Power system analysis
Most practical power system problems are solved by using steady state analytical methods. These methods make the assumption that circuit parameters are linear, bilateral, and constant for constant frequency circuit variables.

When analysing initial values, it is necessary to study the behaviour of a circuit in the transient state. This can be achieved using operational methods.
In some problems, which fortunately are rare, the assumption of linear, bilateral circuit parameters is no longer valid. Such problems are solved using advanced mathematical techniques that are beyond the scope of this article.
1. Circuit Laws (brief descriptions)
In linear, bilateral circuits, there are three basic network laws. These laws apply, regardless of the state of the circuit, and at any particular instant of time.
These laws are the branch, junction and mesh laws, derived from Ohm and Kirchhoff, and are stated below, using steady state a.c. nomenclature.
1.1 Branch law
The current I in a given branch of impedance Z is proportional to the potential difference V appearing across the branch, that is:
![]()
![]()
1.2 Junction law
The algebraic sum of all currents entering any junction (or node) in a network is zero, that is:
![]()
![]()
1.3 Mesh law
The algebraic sum of all the driving voltages in any closed path (or mesh) in a network is equal to the algebraic sum of all the passive voltages (products of the impedances and the currents) in the component branches, that is:
![]()
![]()
Alternatively, the total change in potential around a closed loop is zero.
2. Circuit Theorems (brief descriptions)
From the above network laws, many theorems have been derived for the rationalization of networks, either to reach a quick, simple, solution to a problem or to represent a complicated circuit by an equivalent.
Of the many theorems that exist, the three most important are given. These are:
- Superposition Theorem,
- Thévenin’s Theorem and
- Kennelly’s Star/Delta Theorem.
2.1 Superposition Theorem (general network theorem)
The resultant current that flows in any branch of a network due to the simultaneous action of several driving voltages is equal to the algebraic sum of the component currents due to each driving voltage acting alone with the remainder short-circuited.
2.2 Thévenin’s Theorem (active network reduction theorem)
Any active network that may be viewed from two terminals can be replaced by single driving voltage acting in series with single impedance.
The driving voltage is the open-circuit voltage between the two terminals and the impedance is the impedance of the network viewed from the terminals with all sources short-circuited.
2.3 Kennelly’s Star/Delta Theorem (passive network reduction theorem)
Any three-terminal network can be replaced by a delta or star impedance equivalent without disturbing the external network. The formulae relating the replacement of a delta network by the equivalent star network is as follows:
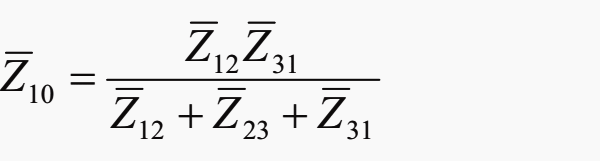
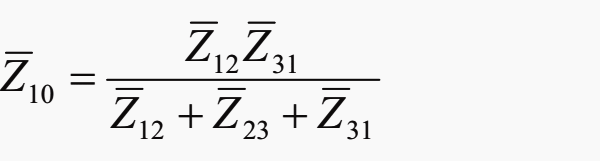
and so on.


The impedance of a delta network corresponding to and replacing any star network is:
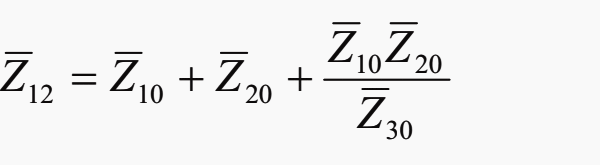
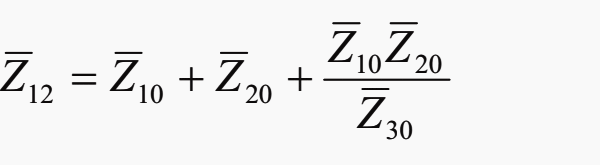
and so on.
3. Network Reduction
The aim of network reduction is to reduce a system to a simple equivalent while retaining the identity of that part of the system to be studied.
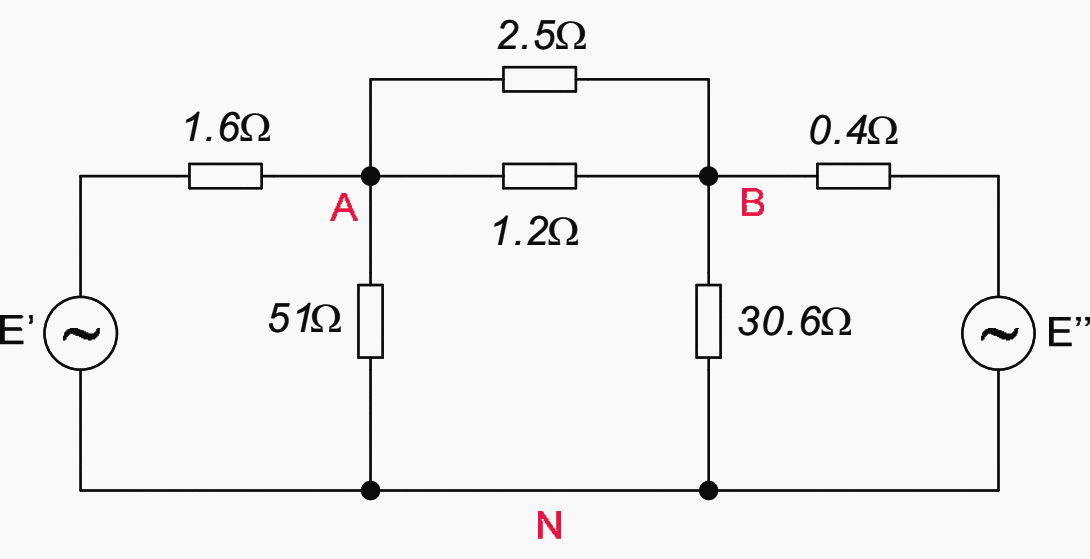
For example, consider the system shown in Figure 2.
The network has two sources E’ and E” , a line AOB shunted by an impedance, which may be regarded as the reduction of a further network connected between A and B, and a load connected between O and N.
Proceeding, A, B, N, forms a star branch and can therefore be converted to an equivalent delta.




(Since ZNO >> ZAOZBO)
The network is now reduced as shown in Figure 3.

By applying Thévenin’s theorem to the active loops, these can be replaced by a single driving voltage in series with impedance, as shown in Figure 4.


The network shown in Figure 2 is now reduced to that shown in Figure 3.12 with the nodes A and B retaining their identity. Further, the load impedance has been completely eliminated.
The network shown in Figure 5 may now be used to study system disturbances, for example power swings with and without faults.


Most reduction problems follow the same pattern as the example above. The rules to apply in practical network reduction are:
- Decide on the nature of the disturbance or disturbances to be studied.
- Decide on the information required, for example the branch currents in the network for a fault at a particular location.
- Reduce all passive sections of the network not directly involved with the section under examination.
- Reduce all active meshes to a simple equivalent, that is, to a simple source in series with a single impedance.
In certain circuits, for example parallel lines on the same towers, there is mutual coupling between branches. Correct circuit reduction must take account of this coupling.
Three cases are of interest. These are:
- Case a: two branches connected together at their nodes
- Case b: two branches connected together at one node only
- Case c: two branches that remain unconnected
Considering each case in turn:
Case a
Consider the circuit shown in Figure 6(a).
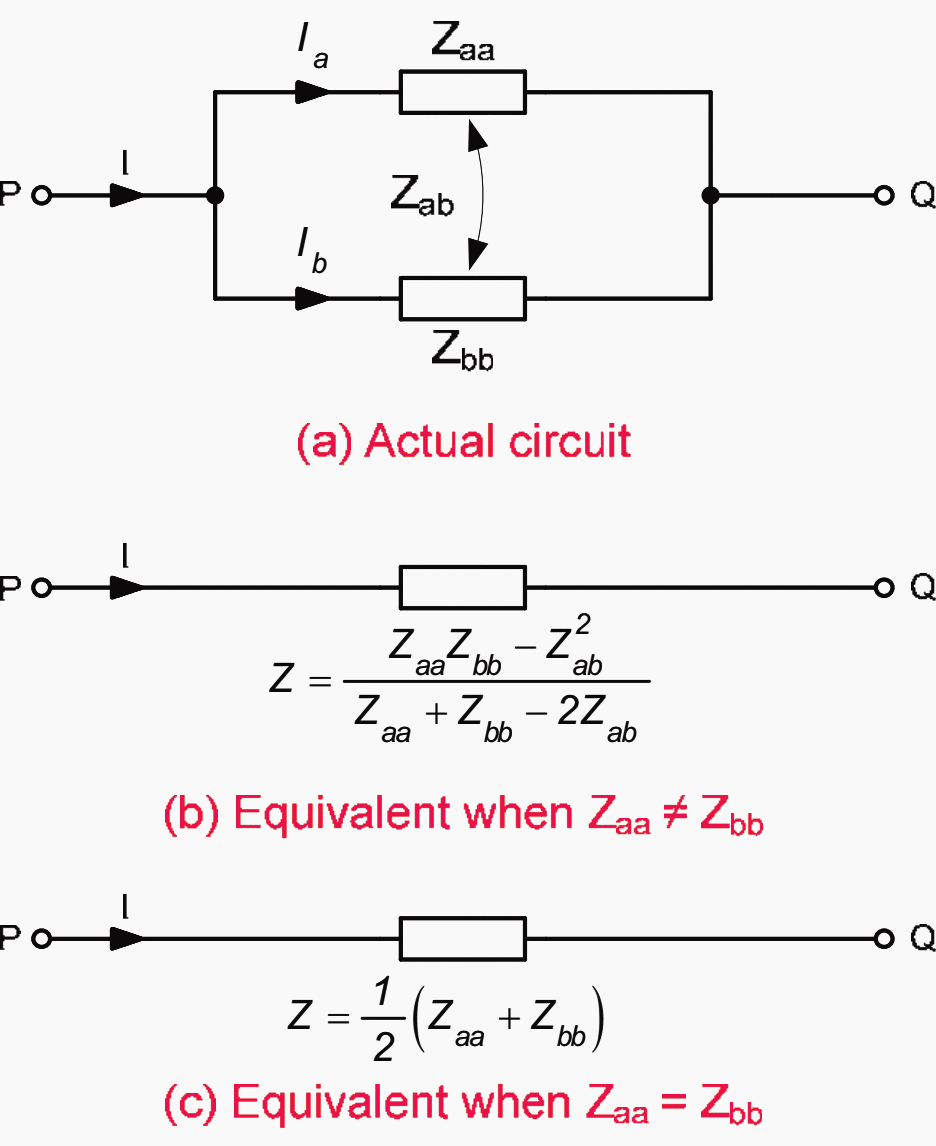

The application of a voltage V between the terminals P and Q gives:
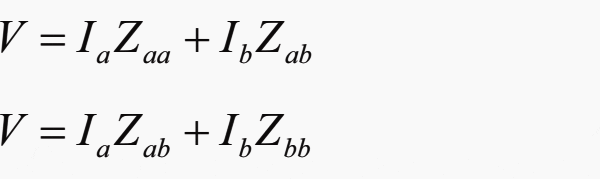
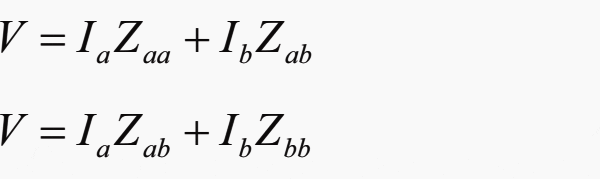
where: Ia and Ib are the currents in branches a and b, respectively and I = Ia + Ib , the total current entering at terminal P and leaving at terminal Q.
Solving for Ia and Ib:
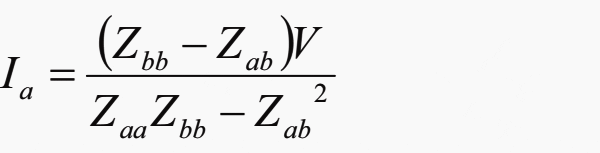
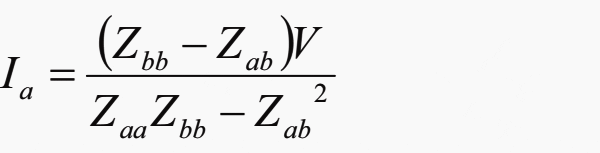
from which
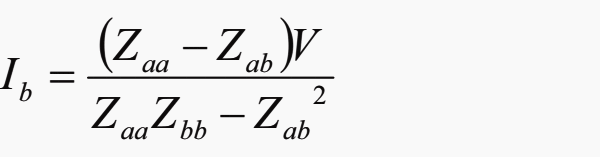
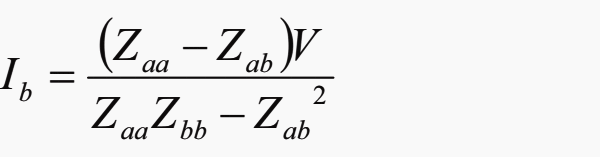
and


so that the equivalent impedance of the original circuit is (see Figure 6b):
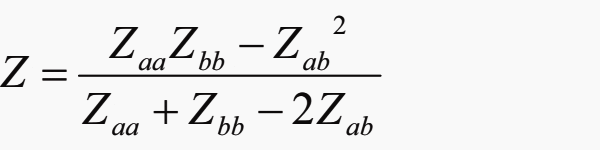
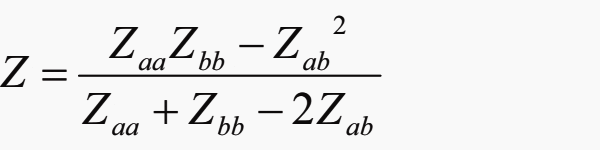
and, if the branch impedances are equal, the usual case, then (see Figure 6c):
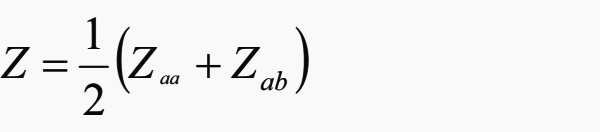
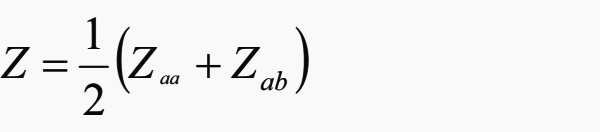
Case b
Consider the circuit in Figure 7(a)


The assumption is made that an equivalent star network can replace the network shown. From inspection with one terminal isolated in turn and a voltage V impressed across the remaining terminals it can be seen that:


Solving these equations gives (see Figure 7b):
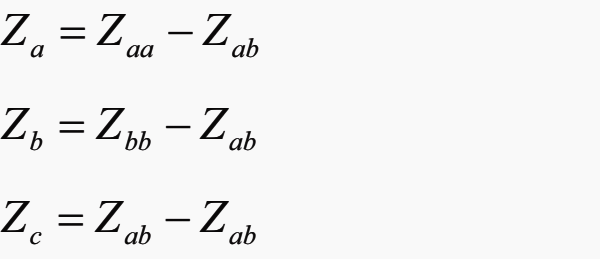
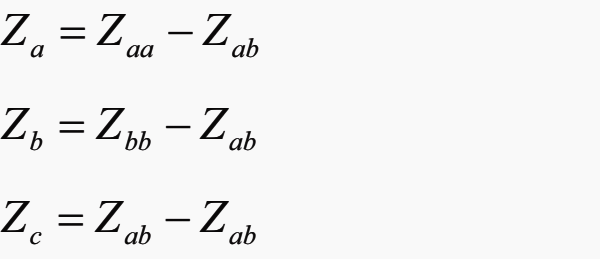
Case c
Consider the four-terminal network given in Figure 8(a), in which the branches 11′ and 22′ are electrically separate except for a mutual link.
The equations defining the network are:
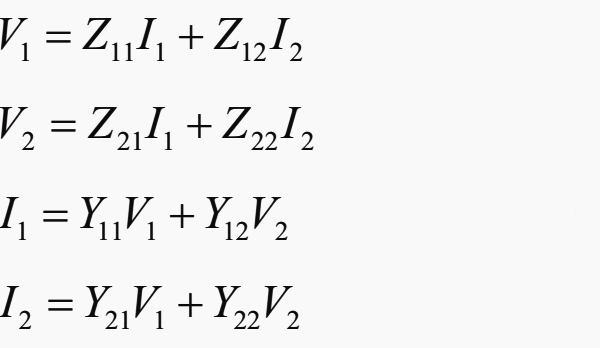
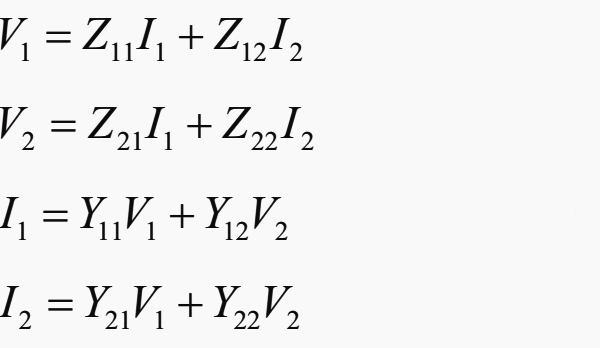
where Z12 = Z21 and Y12 = Y21, if the network is assumed to be reciprocal. Further, by solving the above equations it can be shown that:
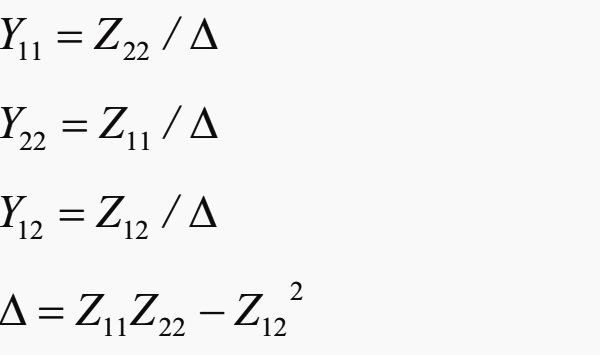
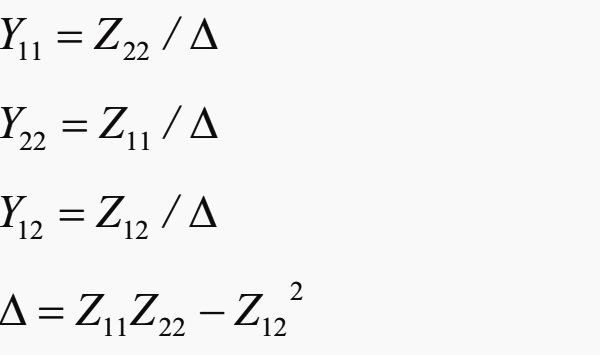
There are three independent coefficients, namely Z12, Z11, Z22 so the original circuit may be replaced by an equivalent mesh containing four external terminals, each terminal being connected to the other three by branch impedances as shown in Figure 7(b).
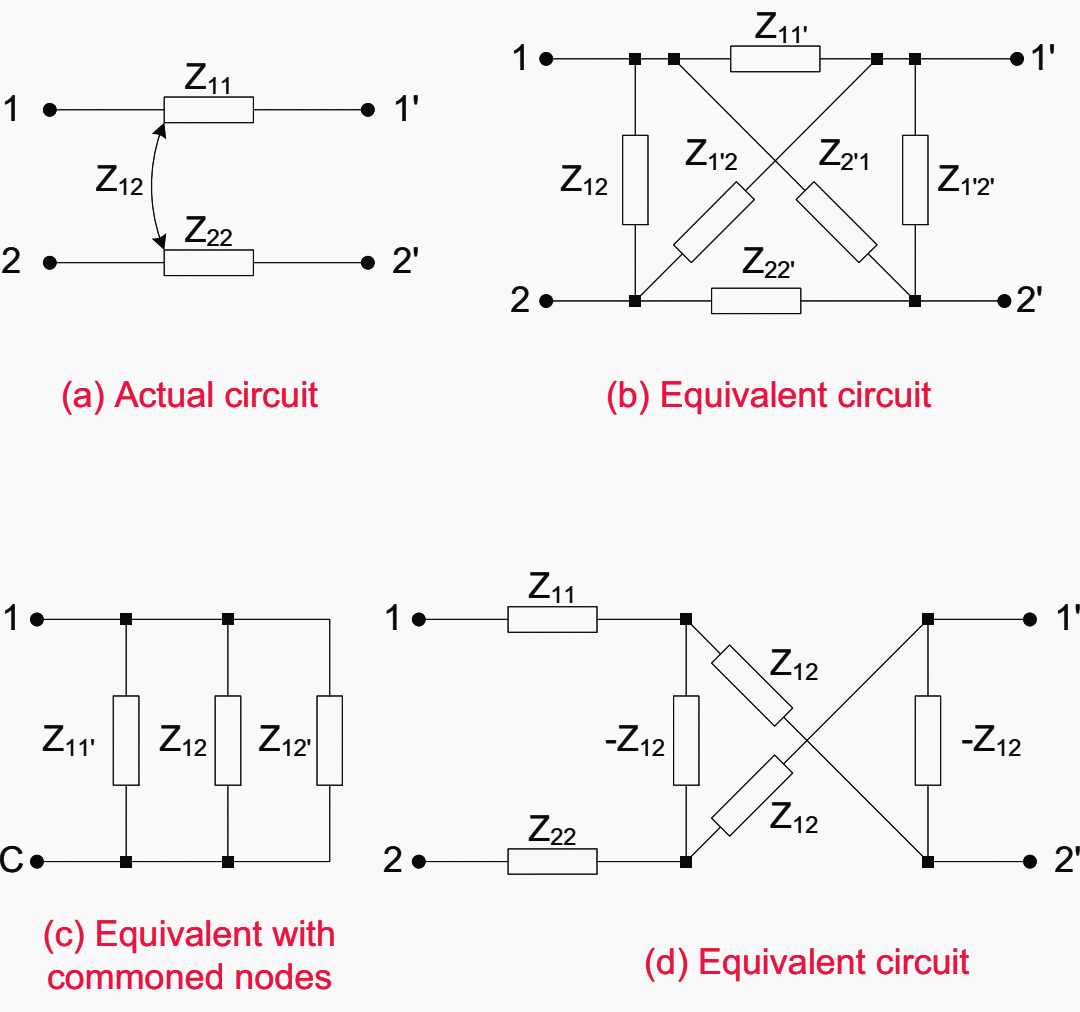

In order to evaluate the branches of the equivalent mesh, let all points of entry of the actual circuit be commoned except node 1 of circuit 1, as shown in Figure 7(c). Then all impressed voltages except V1 will be zero and:


If the same conditions are applied to the equivalent mesh, then:
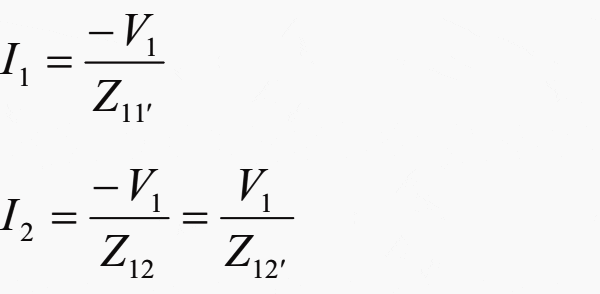
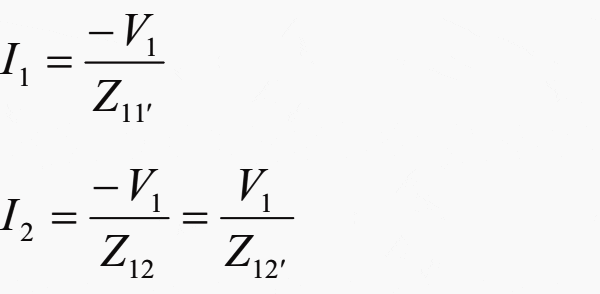
These relations follow from the fact that the branch connecting nodes 1 and 1′ carries current I1 and the branches connecting nodes 1 and 2′ and 1′ and 2 carry current I2. This must be true since branches between pairs of commoned nodes can carry no current.
By considering each node in turn with the remainder commoned, the following relationships are found:
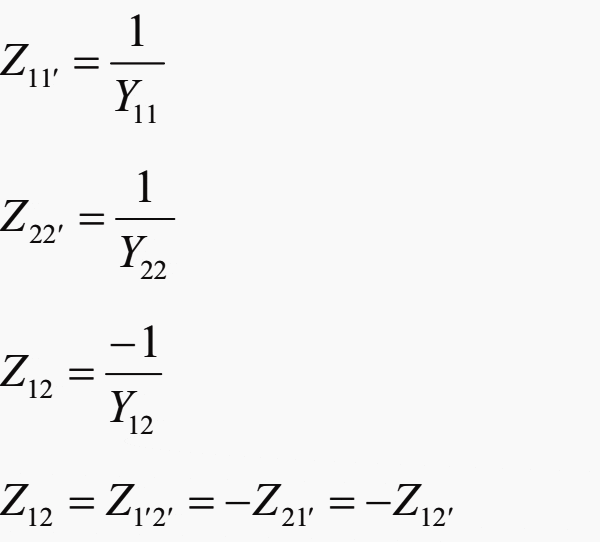
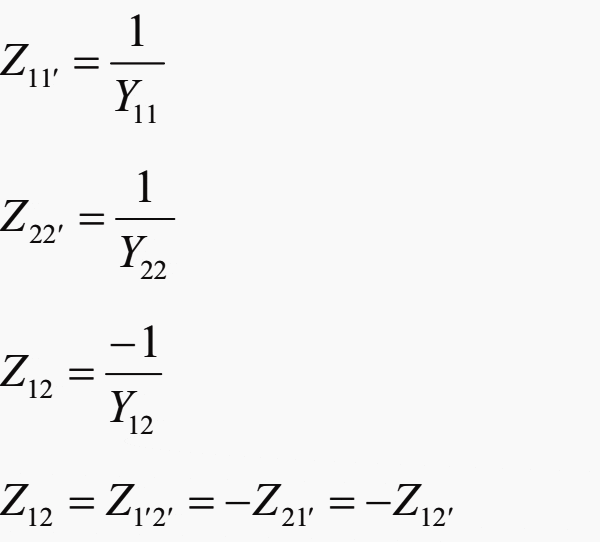
Hence:
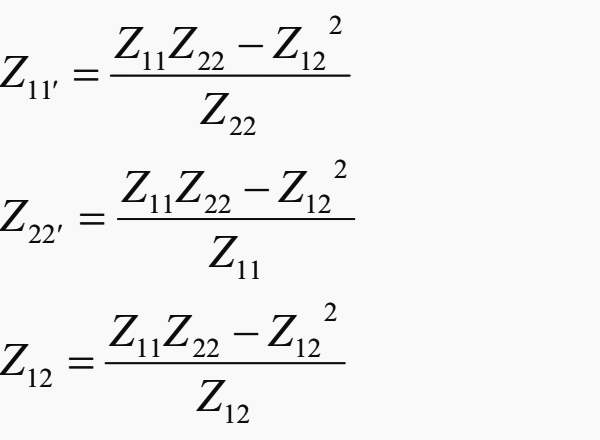
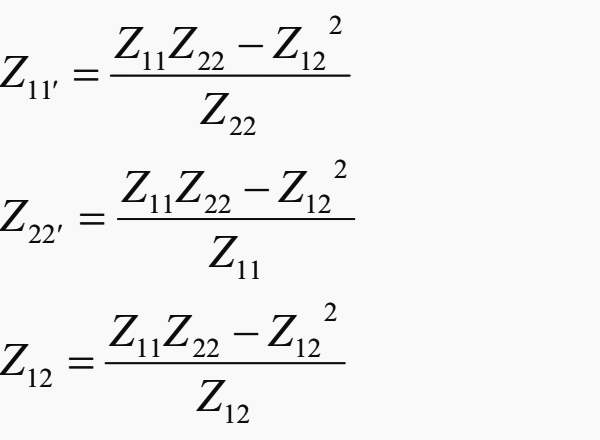
A similar but equally rigorous equivalent circuit is shown in Figure 7(d). This circuit follows from the reasoning that since the self-impedance of any circuit is independent of all other circuits it need not appear in any of the mutual branches if it is lumped as a radial branch at the terminals.
So putting Z11 and Z22, equal to zero in the last equation, defining the equivalent mesh in Figure 7(b), and inserting radial branches having impedances equal to Z11 and Z22 in terminals 1 and 2, results in Figure 7(d).
Source: Network protection & automation guide by Alstom Grid











Features to be considered in undergrounding overhead Power lines in distribution